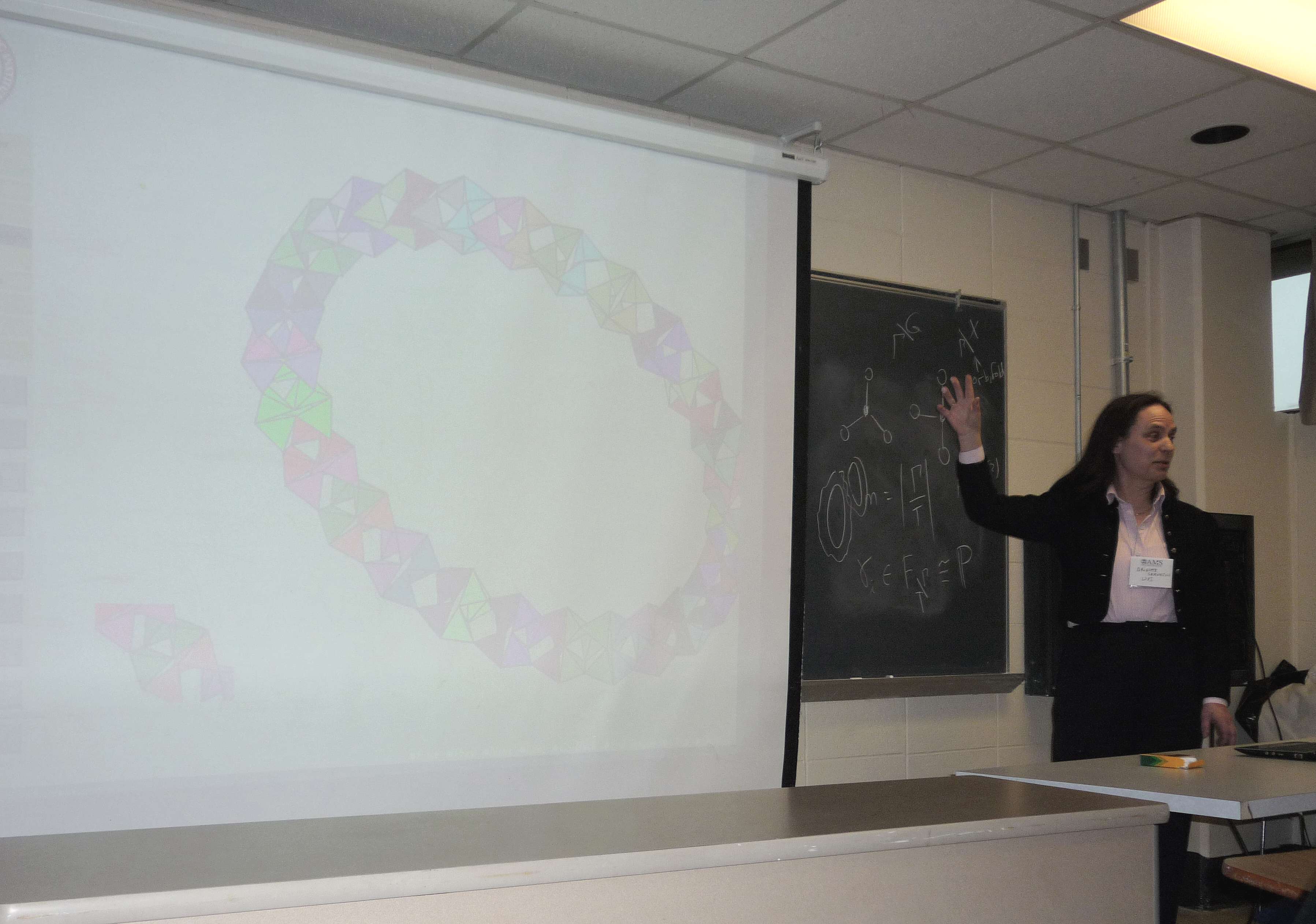

1098-05-105Brigitte Servatius*(bservat@wpi.edu), Mathematical Sciences, WPI, 100 Institute Road,Worcester, MA 01609-2280. Constructing finite zeolites.Preliminary report.In materials chemistry, a zeolite is a crystalline solid formed of units consisting of a silicon (or other tetrahedrally coordinated) atom surrounded by four covalently bonded oxygen atoms. Each oxygen has the opportunity to form another bond, but the bond angles in the unit forbids a bond between the oxygens of the same unit as well as more than one bond between oxygens of different units, so it is common for each unit to bond at the oxygens to four distinct other units. A key feature of zeolites is the presence of relatively large empty regions (pores) within the solid through which other molecules, such as water or hydrocarbons, may pass, so zeolites are useful in many applications as a micro-filter.They are usually modeled as infinite periodic structures. However, the underlying graph of a zeolite is the line graph of a4-regular graph and there are aperiodic and finite examples. The question arises which of these combinatorial examples have geometric realizations as unit distance graphs with non-intersecting tetrahedra in 3-space and whether or not the finite examples possess the key properties of the infinite ones.